
The last steps make use of the polynomial forms of integrals. by the way moment of inertia is defined, this would increase the total moment of inertia. Now take any infinitesimal amount of mass on the circumference and move it to a radius greater than that of the circle.
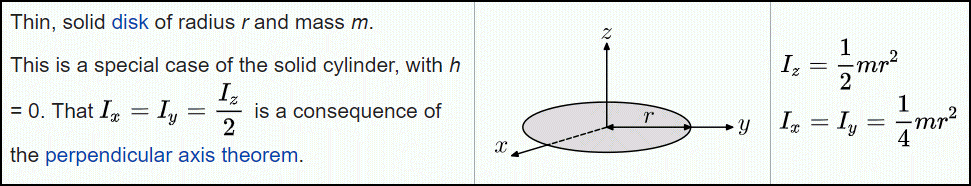
If you take the limiting case of R=0 you get the thin rod expression, and if you take the case where L=0 you get the thin disk expression. Well to prove that each cross section disc has to be a circle, I would start by assuming that it was a circle to start with.
#MOMENT OF INERTIA OF A CIRCLE PROOF PLUS#
This form can be seen to be plausible it you note that it is the sum of the expressions for a thin disk about a diameter plus the expression for a thin rod about its end. Now expressing the mass element dm in terms of z, we can integrate over the length of the cylinder. For any given disk at distance z from the x axis, using the parallel axis theorem gives the moment of inertia about the x axis. This involves an integral from z=0 to z=L.
#MOMENT OF INERTIA OF A CIRCLE PROOF FULL#
Obtaining the moment of inertia of the full cylinder about a diameter at its end involves summing over an infinite number of thin disks at different distances from that axis. The approach involves finding an expression for a thin disk at distance z from the axis and summing over all such disks. The development of the expression for the moment of inertia of a cylinder about a diameter at its end (the x-axis in the diagram) makes use of both the parallel axis theorem and the perpendicular axis theorem. Moment of Inertia: Cylinder About Perpendicular Axis The only difference from the solid cylinder is that the integration takes place from the inner radius a to the outer radius b: Show development of thin shell integral The process involves adding up the moments of infinitesmally thin cylindrical shells. The expression for the moment of inertia of a hollow cylinder or hoop of finite thickness is obtained by the same process as that for a solid cylinder. Substituting gives a polynomial form integral: The mass element can be expressed in terms of an infinitesmal radial thickness dr by Hence, for this problem, dI 1 2r2 dm d I 1 2 r 2 d m. Recall the moment of inertia for a solid cylinder: I 1 2M R2 I 1 2 M R 2. Using the general definition for moment of inertia: Slice up the solid sphere into infinitesimally thin solid cylinders. The parallel axis theorem is used to determine the moment of inertia of composite sections. Moment of Inertia: CylinderThe expression for the moment of inertia of a solid cylinder can be built up from the moment of inertia of thin cylindrical shells. The moment of inertia of an area with respect to any given axis is equal to the moment of inertia with respect to the centroidal axis plus the product of the area and the square of the distance between the 2 axes. Show development of expressions Hollow cylinder case The moments of inertia for the limiting geometries with this mass are: I thin disk diameter = kg m 2 Length L = m,the moments of inertia of a cylinder about other axes are shown. Will have a moment of inertia about its central axis: I central axis = kg m 2 3 provides the moment of inertia and section modulus formula for common geometrical shapes.Parallel Axis Theorem Moment of Inertia: Cylinder

In SI unit systems the unit of Section Modulus is m 3 and in US unit system inches 3. Section modulus is denoted by “Z” and mathematically expressed as Z=I/y Section modulus of a section is defined as the ratio of moment of inertia (I) to the distance (y) of extreme fiber from the neutral axis in that section. M.O.I relative to the origin, J o I x + I y r 4 + r 4 r 4. Now to determine the semicircle’s moment of inertia we will take the sum of both the x and y-axis. Now this gives us I x I y r 4 (A o) R 2 (r 2) R 2. The larger the moment of inertia, the greater is the moment of resistance against bending. Here the M.O.I will be half the moment of inertia of a full circle. Bending stresses are inversely proportional to the Moment of Inertia.

Area moment of inertia is the property of a geometrical shape that helps in the calculation of stresses, bending, and deflection in beams. Parallel Axis Theorem, Moment of Inertia Proof.Mass moment of inertia provides a measure of an object’s resistance to change in the rotation direction.


 0 kommentar(er)
0 kommentar(er)
